Pro.ID21543 TitleHuman Knot Title链接http://10.20.2.8/oj/exercise/problem?problem_id=21543 AC0 Submit0 Ratio- 时间&空间限制描述A classic ice-breaking exercise is for a group of n people to form a circle and then arbitrarily join hands with one another. This forms a "human knot" since the players' arms are most likely intertwined. The goal is then to unwind the knot to form a circle of players with no arms crossed. We now adapt this game to a more general and more abstract setting where the physical constraints of the problem are gone. Suppose we represent the initial knot with a 2-regular graph inscribed in a circle (i.e., we have a graph with n vertices with exactly two edges incident on each vertex). Initially, some edges may cross other edges and this is undesirable. This is the "knot" we wish to unwind. A "move" involves moving any vertex to a new position on the circle, keeping its edges intact. Our goal is to make the fewest possible moves such that we obtain one n-sided polygon with no edge-crossings remaining. For example, here is a knot on 4 vertices inscribed in a circle, but two edges cross each other. By moving vertex 4 down to the position between 2 and 3, a graph without edge-crossings emerges. This was achieved in a single move, which is clearly optimal in this case. 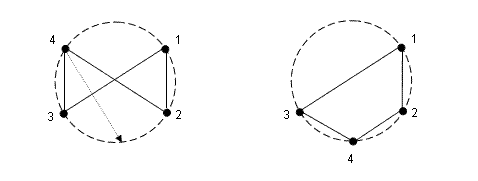 When n is larger, things may not be quite as clear. Below we see a knot on 6 vertices. We might consider moving vertex 4 between 5 and 6, then vertex 5 between 1 and 2, and finally vertex 6 between 3 and 4; this unwinds the knot in 3 moves. 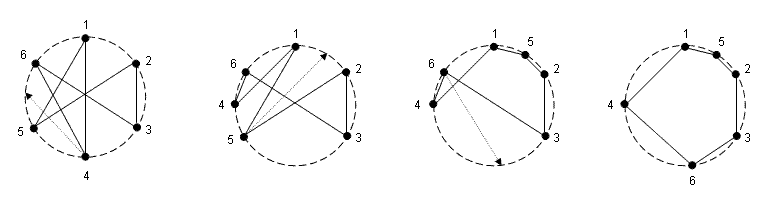 But clearly we can unwind the same knot in only two moves: 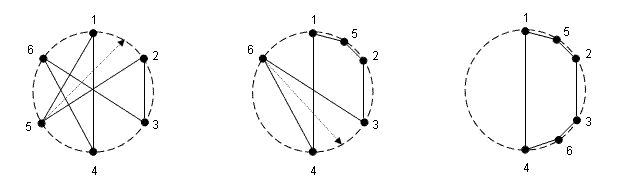 输入The input consists of a number of cases. Each case starts with a line containing the integer n (3 ≤ n ≤ 500), giving the number of vertices of the graph. The vertices are labelled clockwise from 1 to n. Each of the next n lines gives a pair of neighbors, where line i (1 ≤ i ≤ n) specifies the two vertices adjacent to vertex i. The input is terminated by n = 0. 输出Description A classic ice-breaking exercise is for a group of n people to form a circle and then arbitrarily join hands with one another. This forms a "human knot" since the players' arms are most likely intertwined. The goal is then to unwind the knot to form a circle of players with no arms crossed. We now adapt this game to a more general and more abstract setting where the physical constraints of the problem are gone. Suppose we represent the initial knot with a 2-regular graph inscribed in a circle (i.e., we have a graph with n vertices with exactly two edges incident on each vertex). Initially, some edges may cross other edges and this is undesirable. This is the "knot" we wish to unwind. A "move" involves moving any vertex to a new position on the circle, keeping its edges intact. Our goal is to make the fewest possible moves such that we obtain one n-sided polygon with no edge-crossings remaining. For example, here is a knot on 4 vertices inscribed in a circle, but two edges cross each other. By moving vertex 4 down to the position between 2 and 3, a graph without edge-crossings emerges. This was achieved in a single move, which is clearly optimal in this case.  When n is larger, things may not be quite as clear. Below we see a knot on 6 vertices. We might consider moving vertex 4 between 5 and 6, then vertex 5 between 1 and 2, and finally vertex 6 between 3 and 4; this unwinds the knot in 3 moves.  But clearly we can unwind the same knot in only two moves:  Input The input consists of a number of cases. Each case starts with a line containing the integer n (3 ≤ n ≤ 500), giving the number of vertices of the graph. The vertices are labelled clockwise from 1 to n. Each of the next n lines gives a pair of neighbors, where line i (1 ≤ i ≤ n) specifies the two vertices adjacent to vertex i. The input is terminated by n = 0. Output For each case, if there is no solution, print "Not solvable." on a line by itself. If there is a solution, print "Knot solvable." on a line by itself, followed by the minimum number of moves required to solve the problem, on a line by itself. Sample Input 6 Sample Output Knot solvable. Source 样例输入6 样例输出Knot solvable. 作者 |